Filters
Question type
Correct Answer

verified
\(\mathbf { u } + \mathbf { v } = \langle 0,3 b , c \rangle ; \mathbf { u } - \mathbf { v } = \langle 2 a , b , 5c \rangle ; 3 \mathbf { u } - \frac { 1 } { 2 } \mathbf { v } = \left\langle \frac { 7 } { 2 } a , \frac { 11 } { 2 } b , 10 c \right\rangle\)
Correct Answer
verified
\(\mathbf { u } + \mathbf { v } = \langle 0,3 b , c \rangle ; \mathbf { u } - \mathbf { v } = \langle 2 a , b , 5c \rangle ; 3 \mathbf { u } - \frac { 1 } { 2 } \mathbf { v } = \left\langle \frac { 7 } { 2 } a , \frac { 11 } { 2 } b , 10 c \right\rangle\)
Question 2
Essay
Find and , given that and .
Correct Answer

verified
Correct Answer
verified
Question 3
Essay
Find a vector that is perpendicular to the plane passing through the three given points.
Correct Answer

verified
\(\langle 8,42,10 \rangle\)
Correct Answer
verified
\(\langle 8,42,10 \rangle\)
Question 4
Essay
Two vectors u and v are given. Find the angle (expressed in degrees) between u and v.
Correct Answer

verified
Correct Answer
verified
Question 5
Essay
Describe the trace of the sphere through (a) the xz-plane in (b) the plane .
Correct Answer

verified
a) circle ...View Answer
Show Answer
Correct Answer
verified
a) circle ...
View Answer
Question 6
Short Answer
Describe the surface represented by the given equation.
Correct Answer

verified
Plane para...View Answer
Show Answer
Correct Answer
verified
Plane para...
View Answer
Question 7
Essay
Determine whether is orthogonal to .
Correct Answer

verified
\(\mathbf { u } \cdot \mathbf { v } = \left( - \frac { 1 } { 2 } \right) ( - 2 ) + ( 2 ) \left( \frac { 1 } { 2 } \right) = 2\) , not orthogonal
Correct Answer
verified
\(\mathbf { u } \cdot \mathbf { v } = \left( - \frac { 1 } { 2 } \right) ( - 2 ) + ( 2 ) \left( \frac { 1 } { 2 } \right) = 2\) , not orthogonal
Question 8
Essay
Given and in the figure, sketch . 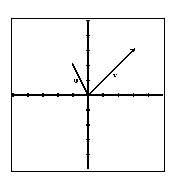
Correct Answer

verified
Correct Answer
verified
Question 9
Essay
Two vectors u and v are given. Find their dot product .
Correct Answer

verified
Correct Answer
verified
Question 10
Short Answer
The lengths of two vectors a and b, and the angle between them, are given. Find the length of their cross product, .
Correct Answer

verified
Correct Answer
verified
Question 11
Essay
Find the area of the parallelogram determined by the given vectors.
Correct Answer

verified
Correct Answer
verified
Question 12
Essay
Find an equation of a sphere with the given radius r and center C. ;
Correct Answer

verified
Correct Answer
verified
Question 13
Essay
Given and , calculate and then resolve into and .
Correct Answer

verified
Correct Answer
verified
Question 14
Essay
A description of a line is given. Find parametric equations for the line. The line perpendicular to the xz-plane that contains the point .
Correct Answer

verified
Correct Answer
verified
Question 15
Essay
Find parametric equations for the line that passes through the points P and Q.
Correct Answer

verified
Correct Answer
verified
Question 16
Essay
Find the vector v with initial point P and terminal point Q. ,
Correct Answer

verified
Correct Answer
verified
Question 17
Essay
Find the area of the parallelogram determined by the given vectors.
Correct Answer

verified
Correct Answer
verified
Question 18
Essay
Given that the forces , and are acting on a point , find the resultant force, magnitude and the additional force required in order for the forces to be in equilibrium.
Correct Answer

verified
The resultant force ...View Answer
Show Answer
Correct Answer
verified
The resultant force ...
View Answer
Question 19
Essay
Find the vector with initial point and terminal point .
Correct Answer

verified
The vector...View Answer
Show Answer
Correct Answer
verified
The vector...
View Answer
Question 20
Essay
Find , and , given that , and .
Correct Answer

verified
Correct Answer
verified
Showing 1 - 20 of 96
Related Exams