A) At the number of rabbits rebounds to 500 .
B) At the number of foxes reaches a maximum of about 2400 .
C) At the population of foxes reaches a minimum of about 30 .
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Newton's Law of Cooling states that the rate of cooling of an object is proportional to the temperature difference between the object and its surroundings. Suppose that a roast turkey is taken from an oven when its temperature has reached and is placed on a table in a room where the temperature is . If is the temperature of the turkey after minutes, then Newton's Law of Cooling implies that This could be solved as a separable differential equation. Another method is to make the change of variable . If the temperature of the turkey is after half an hour, what is the temperature after ? Select the correct answer.
A)
B)
C)
D) none of these
E)
Correct Answer

verified
Correct Answer
verified
Short Answer
Select a direction field for the differential equation from a set of direction fields labeled I-IV.
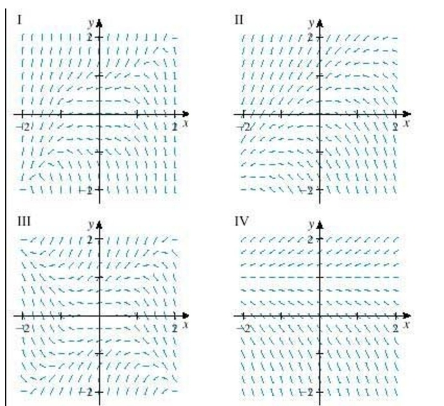
Correct Answer

verified
Correct Answer
verified
Short Answer
Consider a population with constant relative birth and death rates and , respectively, and a constant emigration rate , where and . Then the rate of change of the population at time is modeled by the differential equation where Find the solution of this equation with the rate of change of the population at time that satisfies the initial condition .
Correct Answer

verified
Correct Answer
verified
Short Answer
Suppose that a population develops according to the logistic equation where is measured in weeks. What is the carrying capacity?
Correct Answer

verified
Correct Answer
verified
Short Answer
Newton's Law of Cooling states that the rate of cooling of an object is proportional to the temperature difference between the object and its surroundings. Suppose that a roast turkey is taken from an oven when its temperature has reached and is placed on a table in a room where the temperature is . If is the temperature of the turkey after minutes, then Newton's Law of Cooling implies that This could be solved as a separable differential equation. Another method is to make the change of variable . If the temperature of the turkey is after half an hour, what is the temperature after ?
Correct Answer

verified
Correct Answer
verified
Short Answer
Let be a positive number. A differential equation of the form where is a positive constant, is called a doomsday equation because the exponent in the expression is larger than the exponent 1 for natural growth. An especially prolific breed of rabbits has the growth term . If 7 such rabbits breed initially and the warren has 21 rabbits after 8 months, then when is doomsday?
Correct Answer

verified
Correct Answer
verified
Short Answer
Solve the differential equation.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine whether the differential equation is linear. Select the correct answer.
A) the equation is linear
B) the equation is not linear
Correct Answer

verified
Correct Answer
verified
Short Answer
Solve the differential equation.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine whether the differential equation is linear. Select the correct answer.
A) the equation is linear
B) the equation is not linear
Correct Answer

verified
Correct Answer
verified
Short Answer
Correct Answer

verified
Correct Answer
verified
Short Answer
Let be the performance level of someone learning a skill as a function of the training time . The graph of is called a learning curve. We propose the differential equation as a reasonable model for learning, where is a positive constant. Solve it as a linear differential equation.
Correct Answer

verified
Correct Answer
verified
Short Answer
Correct Answer

verified
Correct Answer
verified
Short Answer
Solve the initial-value problem.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Determine whether the differential equation is linear. Select the correct answer.
A) the equation is linear
B) the equation is not linear
Correct Answer

verified
Correct Answer
verified
Short Answer
One model for the spread of an epidemic is that the rate of spread is jointly proportional to the number of infected people and the number of uninfected people. In an isolated town of 2,000 inhabitants, 130 people have a disease at the beginning of the week and 1,100 have it at the end of the week. How long does it take for of the population to be infected?
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Suppose that a population develops according to the logistic equation where is measured in weeks. What is the carrying capacity? Select the correct answer.
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Short Answer
Solve the differential equation.
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Which equation does the function satisfy?
A)
B)
C)
D)
E)
Correct Answer

verified
Correct Answer
verified
Showing 121 - 140 of 160
Related Exams