Filters
Question type
Study Flashcards

Solve the inequality. Graph the solution set and write the solution set in interval notation. - 2x−6<1\frac { 2 } { x - 6 } < 1 Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { 2 } { x - 6 } < 1 A) (-\infty, 6) B) ( 6,8 ) C) ( - \infty , 6 ) \cup ( 8 , \infty ) D) ( - \infty , 6 ] \cup [ 8 , \infty )


A) (−∞,6) (-\infty, 6)
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { 2 } { x - 6 } < 1 A) (-\infty, 6) B) ( 6,8 ) C) ( - \infty , 6 ) \cup ( 8 , \infty ) D) ( - \infty , 6 ] \cup [ 8 , \infty )
B) (6,8) ( 6,8 )
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { 2 } { x - 6 } < 1 A) (-\infty, 6) B) ( 6,8 ) C) ( - \infty , 6 ) \cup ( 8 , \infty ) D) ( - \infty , 6 ] \cup [ 8 , \infty )
C) (−∞,6) ∪(8,∞) ( - \infty , 6 ) \cup ( 8 , \infty )
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { 2 } { x - 6 } < 1 A) (-\infty, 6) B) ( 6,8 ) C) ( - \infty , 6 ) \cup ( 8 , \infty ) D) ( - \infty , 6 ] \cup [ 8 , \infty )
D) (−∞,6]∪[8,∞) ( - \infty , 6 ] \cup [ 8 , \infty )
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { 2 } { x - 6 } < 1 A) (-\infty, 6) B) ( 6,8 ) C) ( - \infty , 6 ) \cup ( 8 , \infty ) D) ( - \infty , 6 ] \cup [ 8 , \infty )

Correct Answer

verifed

verified

Solve the inequality. Graph the solution set and write the solution set in interval notation. - −3−3x−7>0\frac { - 3 } { - 3 x - 7 } > 0 Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { - 3 } { - 3 x - 7 } > 0 A) ( 0 , \infty ) B) \left( - \infty , \frac { 7 } { 3 } \right) C) \left( - \frac { 7 } { 3 } , \infty \right) D) \left( - \infty , - \frac { 3 } { 7 } \right)


A) (0,∞) ( 0 , \infty )
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { - 3 } { - 3 x - 7 } > 0 A) ( 0 , \infty ) B) \left( - \infty , \frac { 7 } { 3 } \right) C) \left( - \frac { 7 } { 3 } , \infty \right) D) \left( - \infty , - \frac { 3 } { 7 } \right)
B) (−∞,73) \left( - \infty , \frac { 7 } { 3 } \right)
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { - 3 } { - 3 x - 7 } > 0 A) ( 0 , \infty ) B) \left( - \infty , \frac { 7 } { 3 } \right) C) \left( - \frac { 7 } { 3 } , \infty \right) D) \left( - \infty , - \frac { 3 } { 7 } \right)
C) (−73,∞) \left( - \frac { 7 } { 3 } , \infty \right)
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { - 3 } { - 3 x - 7 } > 0 A) ( 0 , \infty ) B) \left( - \infty , \frac { 7 } { 3 } \right) C) \left( - \frac { 7 } { 3 } , \infty \right) D) \left( - \infty , - \frac { 3 } { 7 } \right)
D) (−∞,−37) \left( - \infty , - \frac { 3 } { 7 } \right)
Solve the inequality. Graph the solution set and write the solution set in interval notation. - \frac { - 3 } { - 3 x - 7 } > 0 A) ( 0 , \infty ) B) \left( - \infty , \frac { 7 } { 3 } \right) C) \left( - \frac { 7 } { 3 } , \infty \right) D) \left( - \infty , - \frac { 3 } { 7 } \right)

Correct Answer

verifed

verified

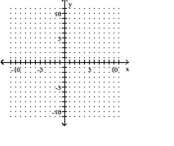
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x2+1f(x) =x^{2}+1 Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x^{2}+1 A) vertex ( - 1,0 ) ; axis x = - 1 B) vertex ( 0 , - 1 ) ; axis x = 0 C) vertex ( 0,1 ) ; axis x = 0 D) vertex ( 1,0 ) ; axis x = 1


A) vertex (−1,0) ( - 1,0 ) ; axis x=−1x = - 1
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x^{2}+1 A) vertex ( - 1,0 ) ; axis x = - 1 B) vertex ( 0 , - 1 ) ; axis x = 0 C) vertex ( 0,1 ) ; axis x = 0 D) vertex ( 1,0 ) ; axis x = 1
B) vertex (0,−1) ( 0 , - 1 ) ; axis x=0x = 0
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x^{2}+1 A) vertex ( - 1,0 ) ; axis x = - 1 B) vertex ( 0 , - 1 ) ; axis x = 0 C) vertex ( 0,1 ) ; axis x = 0 D) vertex ( 1,0 ) ; axis x = 1
C) vertex (0,1) ( 0,1 ) ; axis x=0x = 0
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x^{2}+1 A) vertex ( - 1,0 ) ; axis x = - 1 B) vertex ( 0 , - 1 ) ; axis x = 0 C) vertex ( 0,1 ) ; axis x = 0 D) vertex ( 1,0 ) ; axis x = 1
D) vertex (1,0) ( 1,0 ) ; axis x=1x = 1
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =x^{2}+1 A) vertex ( - 1,0 ) ; axis x = - 1 B) vertex ( 0 , - 1 ) ; axis x = 0 C) vertex ( 0,1 ) ; axis x = 0 D) vertex ( 1,0 ) ; axis x = 1

Correct Answer

verifed

verified

Use the square root property to solve the equation. - x2=2x ^ { 2 } = 2


A) 2\sqrt { 2 }
B) −2,2- \sqrt { 2 } , \sqrt { 2 }
C) 1
D) 4

Correct Answer

verifed

verified

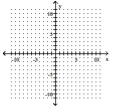
Graph the function. Find the vertex. - G(x) =3(x−3) 2−1G(x) =3(x-3) ^{2}-1 Graph the function. Find the vertex. - G(x) =3(x-3) ^{2}-1 A) vertex: ( 1 , - 3 ) B) vertex: ( - 3 , - 1 ) C) vertex: ( 3 , - 1 ) D) vertex: ( - 1,3 )


A) vertex: (1,−3) ( 1 , - 3 )
Graph the function. Find the vertex. - G(x) =3(x-3) ^{2}-1 A) vertex: ( 1 , - 3 ) B) vertex: ( - 3 , - 1 ) C) vertex: ( 3 , - 1 ) D) vertex: ( - 1,3 )
B) vertex: (−3,−1) ( - 3 , - 1 )
Graph the function. Find the vertex. - G(x) =3(x-3) ^{2}-1 A) vertex: ( 1 , - 3 ) B) vertex: ( - 3 , - 1 ) C) vertex: ( 3 , - 1 ) D) vertex: ( - 1,3 )
C) vertex: (3,−1) ( 3 , - 1 )
Graph the function. Find the vertex. - G(x) =3(x-3) ^{2}-1 A) vertex: ( 1 , - 3 ) B) vertex: ( - 3 , - 1 ) C) vertex: ( 3 , - 1 ) D) vertex: ( - 1,3 )
D) vertex: (−1,3) ( - 1,3 )
Graph the function. Find the vertex. - G(x) =3(x-3) ^{2}-1 A) vertex: ( 1 , - 3 ) B) vertex: ( - 3 , - 1 ) C) vertex: ( 3 , - 1 ) D) vertex: ( - 1,3 )

Correct Answer

verifed

verified

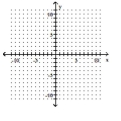
Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =−x2+4x−3f(x) =-x^{2}+4 x-3 Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =-x^{2}+4 x-3 A) B) C) D)


A)
Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =-x^{2}+4 x-3 A) B) C) D)
B)
Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =-x^{2}+4 x-3 A) B) C) D)
C)
Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =-x^{2}+4 x-3 A) B) C) D)
D)
Sketch the graph of the quadratic function by finding the vertex, intercepts, and determining if the graph opens upward or downward. - f(x) =-x^{2}+4 x-3 A) B) C) D)

Correct Answer

verifed

verified

Use the square root property to solve the equation. - (3x+4) 2=5( 3 x + 4 ) ^ { 2 } = 5


A) 5−43,5+43\frac { \sqrt { 5 } - 4 } { 3 } , \frac { \sqrt { 5 } + 4 } { 3 }
B) −3,13- 3 , \frac { 1 } { 3 }
C) 4−53,4+53\frac { 4 - \sqrt { 5 } } { 3 } , \frac { 4 + \sqrt { 5 } } { 3 }
D) −4−53,−4+53\frac { - 4 - \sqrt { 5 } } { 3 } , \frac { - 4 + \sqrt { 5 } } { 3 }

Correct Answer

verifed

verified

Solve. -Find two numbers whose sum is 56 and whose product is as large as possible. [Hint: Let xx and 56−x56 - x be the two numbers. Their product can be described by the function f(x) =x(56−x) f ( x ) = x ( 56 - x ) .]


A) 28 and 84
B) 128\frac { 1 } { 28 } and 1328\frac { 13 } { 28 }
C) 28 and 28
D) 27 and 29

Correct Answer

verifed

verified

Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2x2−4x+5F(x) =2 x^{2}-4 x+5 Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2 x^{2}-4 x+5 A) vertex: ( 1,3 ) x-intercept: ( 0,0 ) , y -intercept: ( 0,5 ) B) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) C) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) D) vertex: ( 1,3 ) x -intercept: ( 5,0 ) , y -intercept: none


A) vertex: (1,3) ( 1,3 )
x-intercept: (0,0) ,y( 0,0 ) , y -intercept: (0,5) ( 0,5 )
Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2 x^{2}-4 x+5 A) vertex: ( 1,3 ) x-intercept: ( 0,0 ) , y -intercept: ( 0,5 ) B) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) C) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) D) vertex: ( 1,3 ) x -intercept: ( 5,0 ) , y -intercept: none

B) vertex: (1,3) ( 1,3 )
xx -intercept: none, yy -intercept: (0,5) ( 0,5 )
Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2 x^{2}-4 x+5 A) vertex: ( 1,3 ) x-intercept: ( 0,0 ) , y -intercept: ( 0,5 ) B) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) C) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) D) vertex: ( 1,3 ) x -intercept: ( 5,0 ) , y -intercept: none

C) vertex: (1,3) ( 1,3 )
xx -intercept: none, yy -intercept: (0,5) ( 0,5 )
Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2 x^{2}-4 x+5 A) vertex: ( 1,3 ) x-intercept: ( 0,0 ) , y -intercept: ( 0,5 ) B) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) C) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) D) vertex: ( 1,3 ) x -intercept: ( 5,0 ) , y -intercept: none

D) vertex: (1,3) ( 1,3 )
xx -intercept: (5,0) ,y( 5,0 ) , y -intercept: none
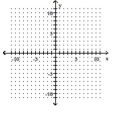
Graph the function. Find the vertex, y-intercept, and x-intercepts (if any) . - F(x) =2 x^{2}-4 x+5 A) vertex: ( 1,3 ) x-intercept: ( 0,0 ) , y -intercept: ( 0,5 ) B) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) C) vertex: ( 1,3 ) x -intercept: none, y -intercept: ( 0,5 ) D) vertex: ( 1,3 ) x -intercept: ( 5,0 ) , y -intercept: none

Correct Answer

verifed

verified

Match the function with its graph. - f(x) =x2−4xf ( x ) = x ^ { 2 } - 4 x


A)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 4 x A) B) C) D)
B)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 4 x A) B) C) D)
C)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 4 x A) B) C) D)
D)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 4 x A) B) C) D)

Correct Answer

verifed

verified

Use the square root property to solve the equation. - 5x2+55=05 x ^ { 2 } + 55 = 0


A) −i11,i11- \mathrm { i } \sqrt { 11 } , \mathrm { i } \sqrt { 11 }
B) −11,11- \sqrt { 11 } , \sqrt { 11 }
C) −11,11- 11,11
D) −11i,11i- 11 \mathrm { i } , 11 \mathrm { i }

Correct Answer

verifed

verified

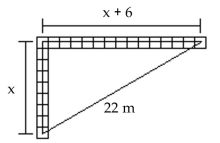
Solve. -Given the diagram shown, approximate to the nearest meter, how many meters of walking distance a person saves by cutting across the lawn instead of walking on the sidewalk. Solve. -Given the diagram shown, approximate to the nearest meter, how many meters of walking distance a person saves by cutting across the lawn instead of walking on the sidewalk. A) 21 \mathrm {~m} B) 33 \mathrm {~m} C) 10 \mathrm {~m} D) 9 \mathrm {~m}


A) 21 m21 \mathrm {~m}
B) 33 m33 \mathrm {~m}
C) 10 m10 \mathrm {~m}
D) 9 m9 \mathrm {~m}

Correct Answer

verifed

verified

Solve. -The hypotenuse of an isosceles right triangle is 6 feet longer than either of its legs. Find the exact length of each side.


A) 6ft,6ft,12ft6 \mathrm { ft } , 6 \mathrm { ft } , 12 \mathrm { ft }
B) 62ft,62ft,(6+62) ft6 \sqrt { 2 } \mathrm { ft } , 6 \sqrt { 2 } \mathrm { ft } , ( 6 + 6 \sqrt { 2 } ) \mathrm { ft }
C) (6+62) ft,(6+62) ft,(12+62) ft( 6 + 6 \sqrt { 2 } ) \mathrm { ft } , ( 6 + 6 \sqrt { 2 } ) \mathrm { ft } , ( 12 + 6 \sqrt { 2 } ) \mathrm { ft }
D) (6+2) ft,(6+2) ft,(12+2) ft( 6 + \sqrt { 2 } ) \mathrm { ft } , ( 6 + \sqrt { 2 } ) \mathrm { ft } , ( 12 + \sqrt { 2 } ) \mathrm { ft }

Correct Answer

verifed

verified

C

Match the function with its graph. - f(x) =x2−2x−8f ( x ) = x ^ { 2 } - 2 x - 8


A)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 2 x - 8 A) B) C) D)
B)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 2 x - 8 A) B) C) D)
C)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 2 x - 8 A) B) C) D)
D)
Match the function with its graph. - f ( x ) = x ^ { 2 } - 2 x - 8 A) B) C) D)

Correct Answer

verifed

verified

D

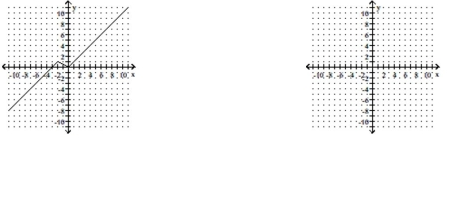
Given the accompanying graph of y = f(x) , sketch the graph of the following. - y=f(x+3) −5y = f ( x + 3 ) - 5 Given the accompanying graph of y = f(x) , sketch the graph of the following. - y = f ( x + 3 ) - 5 y = f ( x ) A) B) C) D) y=f(x) y = f ( x )


A)
Given the accompanying graph of y = f(x) , sketch the graph of the following. - y = f ( x + 3 ) - 5 y = f ( x ) A) B) C) D)

B)
Given the accompanying graph of y = f(x) , sketch the graph of the following. - y = f ( x + 3 ) - 5 y = f ( x ) A) B) C) D)
C)
Given the accompanying graph of y = f(x) , sketch the graph of the following. - y = f ( x + 3 ) - 5 y = f ( x ) A) B) C) D)
D)
Given the accompanying graph of y = f(x) , sketch the graph of the following. - y = f ( x + 3 ) - 5 y = f ( x ) A) B) C) D)

Correct Answer

verifed

verified

Use the square root property to solve the equation. - x2=112x ^ { 2 } = 112


A) 56
B) 14
C) −47,47- 4 \sqrt { 7 } , 4 \sqrt { 7 }
D) 474 \sqrt { 7 }

Correct Answer

verifed

verified

Solve. - x−2+4x−1+3=0x ^ { - 2 } + 4 x ^ { - 1 } + 3 = 0


A) −1,−3- 1 , - 3
B) 1,3
C) 13,1\frac { 1 } { 3 } , 1
D) −13,−1- \frac { 1 } { 3 } , - 1

Correct Answer

verifed

verified

Solve the equation. - x5+4x4=x+4x ^ { 5 } + 4 x ^ { 4 } = x + 4


A) −1,1,−i,i,−4- 1,1 , - i , i , - 4
B) ∅\varnothing
C) 1,i,−41 , i , - 4
D) −1,1,−4- 1,1 , - 4

Correct Answer

verifed

verified

A

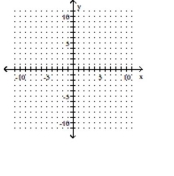
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3x2+5f(x) =3 x^{2}+5 Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3 x^{2}+5 A) vertex ( 5,0 ) ; axis x = 5 B) vertex ( 0,5 ) ; axis x = 5 C) vertex ( 0,5 ) ; axis x = 0 D) vertex ( 0,5 ) ; axis x = 0


A) vertex (5,0) ( 5,0 ) ; axis x=5x = 5
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3 x^{2}+5 A) vertex ( 5,0 ) ; axis x = 5 B) vertex ( 0,5 ) ; axis x = 5 C) vertex ( 0,5 ) ; axis x = 0 D) vertex ( 0,5 ) ; axis x = 0
B) vertex (0,5) ( 0,5 ) ; axis x=5x = 5
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3 x^{2}+5 A) vertex ( 5,0 ) ; axis x = 5 B) vertex ( 0,5 ) ; axis x = 5 C) vertex ( 0,5 ) ; axis x = 0 D) vertex ( 0,5 ) ; axis x = 0
C) vertex (0,5) ( 0,5 ) ; axis x=0x = 0
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3 x^{2}+5 A) vertex ( 5,0 ) ; axis x = 5 B) vertex ( 0,5 ) ; axis x = 5 C) vertex ( 0,5 ) ; axis x = 0 D) vertex ( 0,5 ) ; axis x = 0
D) vertex (0,5) ( 0,5 ) ; axis x=0x = 0
Sketch the graph of the quadratic function. Give the vertex and axis of symmetry. - f(x) =3 x^{2}+5 A) vertex ( 5,0 ) ; axis x = 5 B) vertex ( 0,5 ) ; axis x = 5 C) vertex ( 0,5 ) ; axis x = 0 D) vertex ( 0,5 ) ; axis x = 0

Correct Answer

verifed

verified

Solve. -The cost in millions of dollars for a company to manufacture xx thousand automobiles is given by the function C(x) =4x2−40x+200C ( x ) = 4 x ^ { 2 } - 40 x + 200 . Find the number of automobiles that must be produced to minimize the cost.


A) 5 thousand automobiles
B) 10 thousand automobiles
C) 20 thousand automobiles
D) 100 thousand automobiles

Correct Answer

verifed

verified

Showing 1 - 20 of 242

Related Exams

Exam 1

Review of Real Numbers

490 Questions

Exam 2

Equations, Inequalities, and Problem Solving

332 Questions

Exam 3

Graphing

313 Questions

Exam 4

Solving Systems of Linear Equations

146 Questions

Exam 5

Exponents and Polynomials

304 Questions

Exam 6

Factoring Polynomials

261 Questions

Exam 7

Rational Expressions

327 Questions

Exam 8

More on Functions and Graphs

192 Questions

Exam 9

Inequalities and Absolute Value

148 Questions

Exam 10

Rational Exponents, Radicals, and Complex Numbers

379 Questions

Exam 12

Exponential and Logarithmic Functions

302 Questions

Exam 13

Conic Sections

153 Questions

Exam 14

Sequences, Series, and the Binomial Theorem

201 Questions

Show Answer